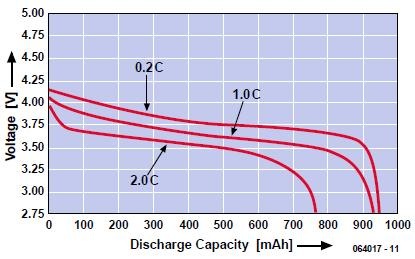
It is not generally known that it is possible to ascertain the extent of charge of a battery with a standard digital voltmeter. It does not apply to all kinds of battery, but it does to, for instance, Lithium-ion batteries. Although there are quite a few different types of Li-ion batteries, it is possible to generalize to a degree. The graphs in the figure (from Panasonic) show clearly that the terminal voltage of the cell drops in direct relation to the diminution of the charge. This means that a simple voltage measurement suffices to determine the state of charge of the battery. Note that the figure shows three graphs each relating to a given load. This means that the output voltage must be measured under load conditions to obtain a satisfactory result. Moreover, the value of the load must be known. Also, the battery must be under load for at least a minute.
There are two ways to proceed. If the load is known and constant as, for instance, in a pocket torch, measure the voltage and read the corresponding charge from the graph. If there is no load, or it is not known or variable, apply a temporary load in the form of a resistor. If the value of this is 20 Ω, for instance, use the upper graph (0.2 C, 180 mA). If a single resistor is used, this will get quite hot, because it has to dissipate 0.66 W, whereas most standard resistors are only rated at 0.25 W to 0.33 W. It is therefore wise to use a number of resistors in parallel, for example, five of 100 Ω each.
To obtain more exact measurements, first draw the graph of your particular battery. Charge it fully and then connect the load, for instance, the five 100 Ω resistors. Measure the output voltage every five minutes and enter the results on an Excel sheet to give a nice curve. If the 5-minute intervals are not exact, enter the real times and choose ‘spread’ as curve. Only this type of sheet can cope with irregular measurement intervals. Moreover, Excel is able to transpose the time on the horizontal (x-) axis into charge. Calculate the current during an interval by dividing the mean voltage (start voltage plus final voltage divided by 2) by the resistance. The charge is the current thus computed times the elapsed time.
The graph shown applies to a battery of 900 mAh.
A current of 0.2 C is then 0.2 x 900 = 180 mA; 1 C is 900 mA; 2 C is 2 x 900 = 1.8 A.
The proposed method is not suitable for NiCd or NiMH batteries, but it is for lead-acid batteries, provided that the temperature is constant. Bear in mind that an old lead-acid battery has a slightly different graph from a new one.
(Elektor Electronics Magazine – 07-08/2006)
 | Download this article (direct link #064017-uk.pdf) |
0 comments: